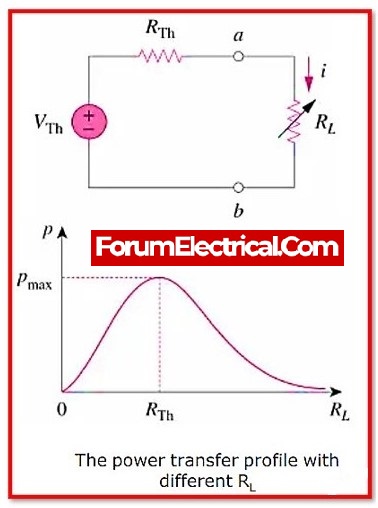
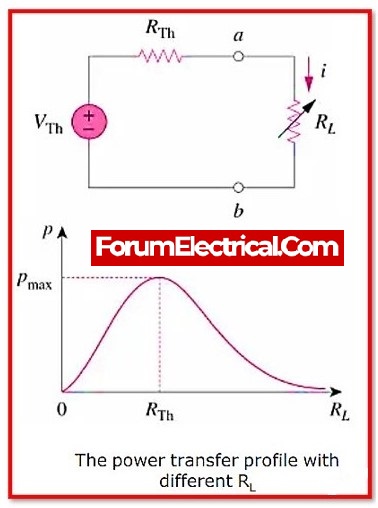
In electrical engineering, the Maximum Power Transfer Theorem states that in a passive, two-port, linear network, the power transmitted to the load is maximised when the load resistance (RL) is equal to the Thevenin equivalent resistance (RTH) of the network. The Thevenin equivalent resistance of a network is the resistance seen looking into the terminals of the network with all sources of voltage removed and the terminals shorted together.
The Maximum Power Transfer Theorem is based on the idea that the power delivered to a load is a function of the load resistance and the voltage and current at the load. When the load resistance is equal to the Thevenin equivalent resistance of the network, the voltage and current at the load are maximized, and the power delivered to the load is also maximized.
The Maximum Power Transfer Theorem is a useful tool for designing electrical circuits and systems, particularly when the goal is to deliver as much power as possible to a load. It allows engineers to determine the optimal load resistance for a given network, ensuring that the power delivered to the load is maximized.
The Maximum Power Transfer Theorem is only applicable to linear, passive two-port networks. It is not applicable to nonlinear networks or to networks with more than two ports. It is also not applicable to active networks, such as those containing amplifiers.
Where,
Current – I
Power – PL
Thevenin’s Voltage – (VTH)
Thevenin’s Resistance – (RTH)
Load Resistance -RL
The power dissipated across load resistor is
PL=I2RL
Substitute I=VTh /RTh+RL in the above equation.
PL=⟮VTh/(RTh+RL)⟯2RL
PL=VTh2{RL/(RTh+RL)2} (Equation 1)
Maximum Power Transfer Conditions:
When the maximum or minimum is reached, the first derivative is zero. So, differentiate Equation 1 with RL and fix it equal to zero.
dPL/dRL=VTh2{(RTh+RL)2×1−RL×2(RTh+RL) / (RTh+RL)4}=0
(RTh+RL)2−2RL(RTh+RL)=0
(RTh+RL)(RTh+RL−2RL)=0
(RTh−RL)=0
RTh=RL or RL=RTh
Therefore, RL=RTh – The condition for maximum power dissipation over the load. That is, if the value of load resistance equals the value of source resistance, i.e., Thevenin’s resistance, then the power distributed across the load is maximised.
The value of Maximum Power Transfer
Substitute RL=RTh & PL=PL,Max in (Equation 1).
PL,Max=VTh2{RTh/ (RTh+RTh)2}
PL,Max=VTh2{RTh/4RTh2}
PL,Max=VTh2/4RTh
PL,Max=VTh2/4RL, since RL=RTh
As a result, the maximum power transferred to load is
PL,Max=VTh2/4RL=VTh2/4RTh
What is the formula for the maximum power transfer theorem?
The condition for maximum power dissipation over the load is
RL=RTh
That is, if the value of load resistance equals the value of source resistance, (i.e.,) Thevenin’s resistance, and the power dissipated across the load is maximised.